7 Top Tips: How to Help Your Child In a Maths Crisis
Updated: Sep 28, 2023

Table of contents
1. Boost Their Confidence so They Expect Great Things
2 Train Them to Become Proactive Problem Solvers
3. Translate Written Maths into Written English
4. Resist Pleas to 'Just Show me the Answer'
5. The Devil is in the Detail
6. Be Selective with Online Resources
7. Dedicate time to Explaining the Secrets of Effective Study
Professional educators will have their own roadmap for becoming awesome. Others, including those short-term homeschoolers who are stressed out by maths will be looking for inspiration.
Anyone who has been following a maths curriculum that they regret buying will, at some point, consider swapping it. My advice would be to resist the temptation. Instead, find out which topics are non-negotiable and double down on them. In the extreme, that can mean outsourcing, but hang fire until you have read this post.
These seven top tips for tutoring maths online will have you teaching like a pro. They are my overarching guidelines and underpin a strategy that transforms suffering students into shining stars. Motivating the student is a large part of it, but this must be backed with substance.
1. Prepare Them Mentally to Expect Great Things
It is a myth that all quick thinkers are just naturally clever
Make it a priority to explain that other seemingly gifted students rarely have special powers. It may look as if the answer just falls from the sky for them, but the skills they possess are teachable. Tell your child your plan is for them to learn these self-same skills and to become every bit as competent and self-assured as their peers. All they have to do is follow advice, put in the hours and believe in themselves.
Get Over the Idea That Rote Learning is a Bad Thing
We can’t really DO maths until we can string ideas together, and we can't really string ideas together until we have retained some useful facts. Rote learning is only bad if we try to memorise material instead of understanding it. The two are not mutually exclusive; the trick is to find a happy medium.
If they are resistant to memorising facts take time out of the lesson and learn it with them. It will save you time in the long run. Be specific and take time to explain how and why those things are relevant. If you yourself are allergic to maths the advice given here should help put you at ease. In future posts I will be fleshing out the bones, so do come back.
View Mental Maths as Agility Training.
I cannot stress this enough. It is so often written off by students as outdated and yet, without it, they are going nowhere. The true value of maths in school is in training us how to recognise patterns. What better starting place than arithmetic. Mental maths is simply like practising the scales when preparing to play at a musical concert.
For years I had wondered why the older students were not competent in mental maths. I finally found the answer when I took on some Primary (Junior) School students. In fact, mental maths is the life-blood of maths education at that age. But after Primary school, students simply fall out of practice and do not feel motivated to use it.
Almost without exception, the majority of older students I had taught had kept their mental maths prowess a very well-hidden secret! I was fascinated to ask them in which grade they thought they were in when they learnt their times tables.
Of course, they had no idea, but it gave me a chance to demonstrate that it was actually in ,Grade 3/Year 4! With this, I had created some friendly competition in them with their younger selves. It was much easier for them to psych themselves up to regaining a Primary/Junior school skill than to 'learn something they don't even have to do at school!' In case I offend any school teachers, it is not my belief, it is their perception!
If your child or student is still not persuaded, you might point out how awkward social situations can be if you can't multiply or divide in your head. They can relate well to that; especially if we paint a mental picture of them out with their friends. All in a good cause!
Encourage Them to Recall Info Rather Than Look It Up
Begin lessons by asking the student what was covered in the last lesson and what they remember. Explain to them that all your sessions will begin this way, going forward because: 'Students who make more effort to recall information have been shown to obtain better results, regardless of whether or not individual retrieval attempts are successful'.
With luck, they may take this on board and use it in other situations. Most students are not lazy. However, they are so used to reaching for the calculator or opening a textbook for use as a cheat sheet it doesn't occur to them to test whether they actually can remember anything.
Show by example how easy problem solving can be, and how much time can be saved when you know your onions. During lessons, create opportunities to demonstrate the benefits of instant recall. Draw attention to how simple that task was for you, because you had the relevant facts in your head already.
My favourite trick is to find a multiplication they don't know and ask them that same one six or seven times during one lesson. The first two or three times they usually recalculate - even though it is the same question. Thankfully, they see it as a bit of fun. It is. And yet it opens their eyes to the fact they just recalled something they 'don't know'. Sometimes they just can't bring themselves to say the correct answer, they still want to say "I don't know". It is so ingrained in them. At least it makes us laugh together.
^ Back to TABLE OF CONTENTS
2. Train them to Become Proactive Problem Solvers
Students lacking in confidence can feel like a rabbit caught in the headlights when you ask them a direct question. In one-to-one situations that will be fairly often! However, once they recognise their performance in basic math is inextricably tied to their performance in mental maths they will make progress and start to believe in themselves. Finally, they get to enjoy the subject.
Learn To Live Without The Calculator - It's Liberating
Before I explain my reasoning here, let's acknowledge that technology in maths is a wonderful thing. I love technology. My concern is only for students who don't realise that over-use of a calculator is impeding their progress at a larger scale.
Often I devote one lesson or two half lessons to a crash course in mental maths. Going over the whole skill set reminds them of what they once knew. That is the beauty of tutoring. You have the freedom to take the lesson down a different rabbit hole every now and again.
I let them see me doing mental maths first, pushing the narrative that life is very simple when you don’t need to keep stopping to look for your calculator. By seeing me do it I mean speaking it all out loud and writing it down in their notebook for future reference.
It is challenging to get them to join in initially, but well worth the effort. By the way, I am a big fan of division by hand because students who don't have that skill seem to suffer more than others. That will be the title of another post!
So often they think mental maths is simply carrying out calculations in their head instead of writing it down on paper. No wonder they can't get excited about it. They don't realise mental maths is primarily about taking safe shortcuts.
Mental maths is an area where homework would have minimal success. It would be like sending a learner driver out to practice alone and then reviewing the dash cam recording with them a week later.
Have them answer a question without the calculator and afterwards ask them to use their calculator to see whether they get the same answer both ways. They like that, and are always impressed when the calculator answer matches their first answer. Talk about putting the cart before the horse!
Enumerate The Important Mental Maths Skills
Here is my list of non-negotiables: multiplication and division by hand, divisibility rules, doubling and halving, recognition of prime numbers, subtraction by counting up, prime factorisation, H.C.F. and L.C.M, fractions decimals and percentage equivalents and fractions of amounts.
If you are not good at mental maths yourself, then you will need to fix that first! In this section you will learn why these guys are in my top 10. If you are already a convert you can easily skip this and move on to the next section.
Multiplication and division by hand
Have them do multiplications and divisions by hand automatically unless you say otherwise. This will provide opportunities for them to learn which calculations are easier by hand and which are not. It For example, 5/7 of 49 has a whole number answer whereas 5/7of 17.21 does not.
Many teenagers who still don't know their tables argue they have got by without them so far and therefore they don't really need them. However, the real value of knowing times tables is that we can more easily work backwards. How can we simplify 48/56 if we don't know our tables backwards, for example? The same problem arises with ratios and similarity and solving equations, let's say algebra in general
Further, we are also much better at spotting number patterns and answering wordy problems when we know our tables. We have more insight. Students who have had these things explained to them are more open to conforming.
Divisibility Rules
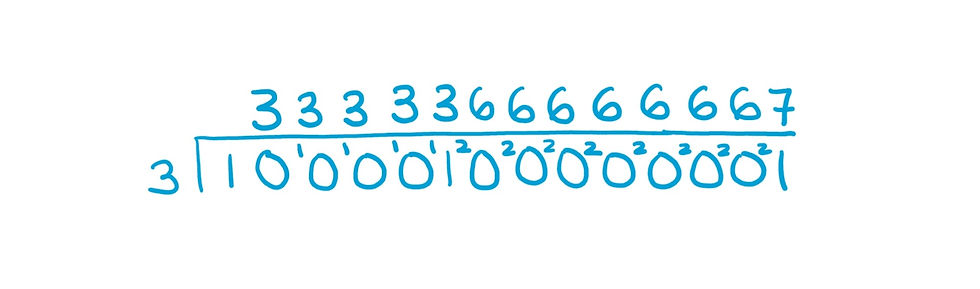
In my view the divisibility rules are more important than the times tables . For example, "Is 10000100000001 in the three times table?" In a flash, the Digit Sum Test tells us "Yes, it is." Without this rule, we would be resigned to actually doing the calculation. You have to admit, there are an awful lot of numbers which are in the 3x table so it's worth learning the test.
Recognising Prime Numbers
Through revising and practising all of the divisibility rules we come to realise that prime numbers are special. Being able to list them has measurable benefits. Prime numbers are indeed our friends and can smash our workload to smithereens.
Doubling and Halving
'Doubling and halving' can be mastered by as little as five or ten minutes of practice once or twice per week. It does need checking though. The main reason for working on this is to make sure your student or child is doing it the easy way.
When you teach this be methodical: for halving work through the integers one y one 1 ÷ 2, 2 ÷ 2, 3 ÷ 2, 4 ÷ 2, , 5 ÷ 2, , 6 ÷ 2 etc. in front of them, and lead them to discover the pattern for themselves. Their sense of accomplishment will spur them on.
Subtraction by counting up
This is THE skill I find most lacking. Many students resort to counting on their fingers even though they freely admit the results they obtain are hit and miss! Failing at subtraction guarantees failure with negative numbers, collecting like terms in algebra, calculating angles in geometry, ... the list is endless.
Discourage the use of mobiles as calculators.
I hate mobile phones being used as calculators, mainly because students don't know what they are doing with them, and every phone seems to have its own quirks and anomalies. What I find is that a student who is using a phone is only half-interested in doing a good job. Besides, they are not able to use their phones during tests or exams and so are missing a trick here.
^ Back to TABLE OF CONTENTS
3. Translate Written Maths into Written English
Common Problem Areas and How to Tackle Them
Some topics you just know are going to cause problems for certain children and yet they will likely report they 'think' they know it. I get it, because often they are able to repeat all of the key phrases for said topic. Only when you attempt questions does it become clear they need help. Whenever you feel you are ahead of the game use that time to tackle one of these topics.
I have focused on some of the commonest repeat offenders below. When I teach these topics I have much greater success when I come at it from an entirely different angle to whoever they learnt from previously. I deliberately leave out the 'familiar' vocabulary until the end so they aren't distracted by it.
Ratios.
Our goal is to have students realise not all ratio questions are equal. Many find it easy enough to share an amount in a given ratio. They become blinded by this and fail to recognise the 'associated' questions such as "Find out the total amount that was shared ...". On the surface, it seems this misconception requires only a simple repair job, but in reality it is often hard work!
A better way to explain a ratio is to involve people and sharing. Say a ratio is similar to a fraction in that the original amount is split into equal parts. The only difference is that some people get more than one share.
Five equal parts and five people means one fifth each. However, five equal parts and only two people means we need a way of writing this to show that the two people did not get the same. Enter the colon.
The maths shorthand for "Dawn got 1 part and Billy got 4 parts" is written as 1 : 4. Much easier than showing the shorthand first and expecting them to understand. Further, by describing ratios in this way it is easier to tackly the reverse type questions.
The difference between a function and an equation
The idea that the graph of y = f(x) is a picture of that function is accepted superficially, but don't dig too deep!
Students are often confused about what to do with something like y = 2x + 3. They try to treat it the same as solving an equation, such as 2x + 3 = 11. In part, this is because y = 2x + 3 may have previously been referred to as an equation when we should call it a function. Tackling this early on will make the whole topic more manageable.
Explaining expressions such as 4 < x < 7 in simple language
Students understand statements such as x > 4, or x < 7 taken separately. But they are doggedly persistent in reading the combined statement 4 < x < 7 as "4 is less than x is less than 7". If you ask them they know they can equally write 4 < x or x > 4 and yet they don't want to equally think it. That is the stumbling block.
Show them how much more useful it is to say that x is allowed to take any value between 4 and 7. Further, in this case, it can't equal either 4 or 7. Emphasise that these symbols are only a form of shorthand and to be taken in context.
Discuss the relation between arithmetic and algebra.
Factorising a number into a product of prime factors and knowing how to use it has many applications. If you are wondering what I am talking about click on this free resource from Dr Frost.
You could be tempted to cut out this topic, yet it is one that will pay for itself over and over again. The prime factorisation of each number is unique and acts effectively as the fingerprint of that number. For example, it can be used to list every ordinary factor of that number. We also use it to find Highest Common Factors (HCF) and Lowest Common Multiples (LCM) and common denominators for adding fractions, and ... ...
As usual, when we deal with small numbers we use simpler tools; ones that we already knew about, including our memory. As we move up the school, the numbers become larger and the simpler techniques fail us. Prime factorisation saves the day.
Crucially, everything we do in algebra follows the same rules as arithmetic. The middle ground between them is substitution. For example. if a = 3 and b = 2, we would be in a sorry state if 2a + 5b did not turn out to equal 16. When students are shown these examples they are usually eager to improve in arithmetic.
Many times I tell a student they have got the right answer and that I am going to show them a different way to do it. They listen politely and then when I ask them what they think of that they say "Yes, I think I get it, but do you mind if I carry on doing it my way?" However, once I explain that my way will allow them to tackle similar questions that are a little bit harder than the one we are on, they appreciate my reasoning.
^ Back to TABLE OF CONTENTS
4. Resist Pleas to 'Just Show me the Answer'.
If you are tutoring a child whose main source of learning is elsewhere there will be times they will ask 'Please, just show me what to write'.
If they have been studying the same topic for some time and still find it impossible they may be feeling panicky. Now, they are desperate to complete an assignment on a topic they really don't understand. Possibly they have concluded they will never be able to get it and that the best option is to stop trying and just go for rote learning.
Their hope of all hopes is that "If I can understand what Dawn is explaining here, I will be able to answer any other questions on this topic. I will be fine." It's tough on them. The majority are just worried they will run out of time.
How many times in my life I have said "Don't worry that we still have 10 questions to answer and there is only half a lesson left ..." What follows depends on the student and their mindset and how much confidence they have in you. Hopefully, you are not the issue!
How do you convince a student they don't quite understand something when they think they do! And, anyway, isn't that counter-intuitive? Sometimes it is unavoidable.
Here, it is better to lay the foundations instead. Explain why and if there is time afterwards, answer some simpler questions.
Better to hold steady and go for quality over quantity. The more confidence they have in their own knowledge the easier it is for them to learn other topics through self-study.
^ Back to TABLE OF CONTENTS
5. Stress That The Devil is in The Detail
Students who are less confident in maths may view a screen as a photo, even the text. They will pick up a few keywords and guess the question - blocking things out to avoid information overload. This is fatal for maths.
Working together helps the student have more patience with themselves and can mark a turning point. I have found it helps for students to keep a 'vocabulary' book similar to the ones they create when learning a foreign language.
Ears need training too. I once had to teach a student on WhatsApp Since there was no screen share option we practised mental maths, Q and A style. What a riot! We were both in fits because we found she couldn't even hold the question in her head - and they were short questions! She was a normal, intelligent kid so it was no reflection on her.
^ Back to TABLE OF CONTENTS
6. Be Selective with Online Resources
We are literally inundated with online resources. Whilst some are life-savers many are not. When you consider how much time it takes to try out these resources and then to choose which ones fits your lesson exactly you may think twice about techy lessons.
Restrain yourself. For what it is worth, students learn equally well in 'pen and paper' presentations as they do with fancy manipulatives and computer games. It's a fact. That said, some tools and websites are just outstanding.
In no particular order, here are my top ten websites for maths tuition. The last three are subscription sites but do have free facilities too. I use the paid versions.
^ Back to TABLE OF CONTENTS
7. Take Time to Explain the Secrets of Effective Study
Smart studying is a hot topic currently. We have all realised it is beneficial to show students how to study effectively and how to become independent learners. My two 'go to' sources are Jim Kwik and Inner Drive.
Jim Kwik
Find him on Facebook or Instagram or visit his website. He had an accident at the age of five and was famously known as the "boy with the broken brain." Self-taught, he has overcome many challenges to become a world leader, revered by the likes of Oprah Winfrey, and Elon Musk. A truly inspirational character who breaks things down into words of one syllable so the rest of us can follow on.
Inner Drive
These guys provide top quality research-based advice for parents and students alike. They are a mindset coaching company with clients as diverse as Eton and Harrow schools, Manchester United FC, and Pepsi. I recently wrote a post that described a podcast they featured in. The podcast was presented by Craig Barton and highlighted their book entitled "The science of learning: 77 studies every teacher needs to know." The podcast is approximately 90 minutes long. If you want to save time you could read my post which only takes 10 minutes to read. Of course the post is only a synopsis.
This is my attempt to provide insight and support to people teaching maths students who are at a crisis point. Even if your student or child is not in this situation you may still find what I have to say enlightening, but your progress will be that much faster. You will need a different approach. Feel free to contact me if you would like more information on how to move forward.

Kommentare